Biological Noise-Based Logic

Fig. 1

Simple demonstration of the difference between the ways of operation for the brain and a computer

Two more quick demonstrations of the difference between the brain and a computer are these: We can try to multiply the first 100 integer numbers and check how long it takes for a laptop computer, it takes less than a millisecond. And we can try to memorize the string “saenstnsjrmenHixerLöeailarenecltcsrhel” or its rearranged version “Hans Liljenström is an excellent researcher”. The second version is much easier for the brain, while the rearrangement does not matter for the computer. More precisely, the first version is easier for the computer because of the lack of blank characters.

2 The Essential Feature of Noise-Based Brain Logic

Due to the limited space, we only illustrate the most essential feature of brain logic [2], which is that the superposition of orthogonal stochastic spike trains carries the information, and the identification of orthogonal components in the superposition is done by coincidence detection (since the neuron is essentially a coincidence detector). As soon as a spike belonging to a component is detected in the superposition by comparing it to the reference signal of the component, its existence is detected, as apparent from Fig. 2. Thus, though the signals are stochastic, no time averaging is needed for interpretation of the signal, and the error probability decays exponentially with increasing waiting time.

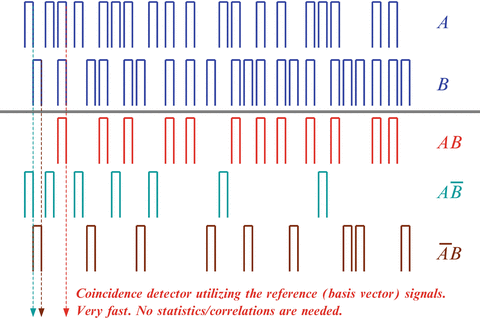
Fig. 2

Demonstration why the brain is so fast notwithstanding that neural spikes are stochastic and occur with low frequency. The existence or non-existence of any of the three orthogonal spike trains in superposition sequences A and B can be quickly observed by coincidence detection, i.e., by neurons

Only gold members can continue reading. Log In or Register to continue