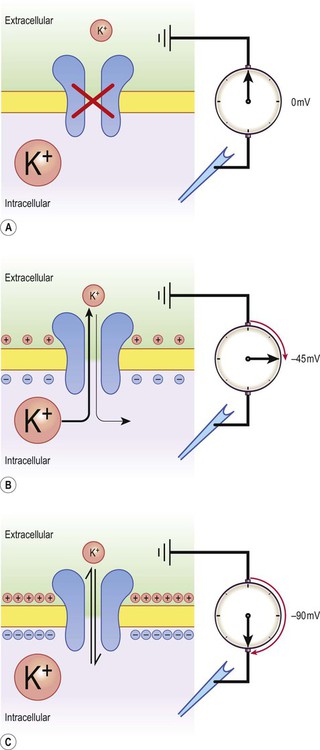
There is a small difference in electrical potential across the plasma membrane of all living cells, the inside usually being slightly negative compared to the outside. This potential difference is referred to as the membrane potential of the cell. It is due to a slight excess of negative charge on the inner face of the plasma membrane and is measured in millivolts (mV). The resting membrane potential can be recorded with an intracellular microelectrode and its value is typically around –70 mV in nerve cells (Fig. 6.1). The transmembrane gradients for sodium and potassium are maintained in the long term by the sodium-potassium exchange pump (‘sodium pump’) which works continuously in the background (Fig. 6.2). The sodium pump is a membrane-bound protein that hydrolyses adenosine triphosphate (ATP) and uses the energy released to move ions across the plasma membrane against their concentration gradients. In each cycle the sodium-potassium pump (or Na+/K+-ATPase) transfers three sodium ions out of the cell and moves two potassium ions into the cell, consuming a single molecule of ATP in the process. In this way, the intracellular potassium concentration is maintained at approximately 140 mM compared to the extracellular concentration of around 3–5.5 mM, representing a potassium gradient of around 35 : 1 (higher on the inside). In contrast, the sodium ion concentration is around 12 mM on the inside and 140 mM on the outside, which equates to a 12 : 1 gradient for sodium ions (higher on the outside). Consider the hypothetical membrane-bound cell depicted in Figure 6.3A. It contains a concentrated solution of potassium salt and is immersed in saline (a solution of sodium chloride). It is important to emphasize that although only potassium (K+) is illustrated in the figure, the intracellular and extracellular fluids contain many different positive and negative ions and are electrically neutral overall. Now suppose that the cell membrane is exclusively permeable to potassium. Since the intracellular concentration is much higher, potassium will passively diffuse out of the cell down its steep concentration gradient (Fig. 6.3B). A slight excess of negative charge will therefore build up on the inner face of the membrane, since each potassium ion that leaves the cell carries a single positive charge. However, this generates a growing electrical field that acts in the opposite direction to the concentration gradient and tends to attract potassium back inside the cell. The net efflux of potassium ions is therefore gradually reduced as the opposing electrical field builds up. Ultimately the rate of potassium efflux (down its concentration gradient) is exactly counterbalanced by potassium influx (down the electrical gradient) and there is no net movement of potassium ions into or out of the cell (Fig. 6.3C). At this equilibrium point the membrane potential is stable and will be slightly more negative on the inside. The potential difference across the membrane at this point is the equilibrium potential for potassium and is around –90 mV. It should be emphasized that this process is very rapid (the equilibrium point is reached almost instantaneously). Under normal physiological conditions, such as constant body temperature, the equilibrium potential for a particular ion is mainly determined by the concentration difference between the inside and outside of the cell. Its value is given by the Nernst equation (Fig. 6.4). This takes into account the size of the transmembrane gradient together with a number of physical and chemical factors including the absolute temperature (measured in Kelvin) and the charge carried by the ion. The resting membrane potential can be calculated by considering the equilibrium potentials for sodium, potassium and chloride ions and factoring in the membrane permeability for each. This information is combined in the Goldman equation (Fig. 6.5) which gives a predicted membrane potential that closely matches recordings from intracellular electrodes. Increasing permeability to a particular ion causes the membrane potential to shift towards the equilibrium potential for that ion. Depending on the starting value of the membrane potential, this may therefore depolarize or hyperpolarize the cell. If the membrane potential is already the same as the equilibrium potential for that particular ion, then opening more ion channels will not alter its value. This concept is illustrated in Figure 6.6 with reference to the chloride channel, which has an equilibrium potential of –65 mV. Figure 6.6A shows the effect of opening additional chloride channels in a membrane that is initially polarized to a value of –60 mV. In this case the membrane is hyperpolarized (from –60 mV to –65 mV). In Figure 6.6B the cell membrane is already at the equilibrium potential for chloride (–65 mV) when the additional chloride channels are opened, so there is no change in membrane voltage (no net ion flux). In Figure 6.6C, the cell membrane starts at a value of –70 mV, which is more negative than the chloride equilibrium potential. Therefore, as the chloride conductance is increased the membrane is depolarized (from –70 mV, towards –65 mV).
Electrical signalling in neurons
The neuron at rest
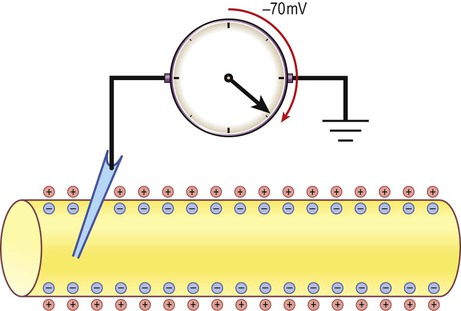
The potential difference across the cell membrane of a typical neuron is around –70 millivolts (negative on the inside).
Origin of the resting membrane potential
The sodium pump
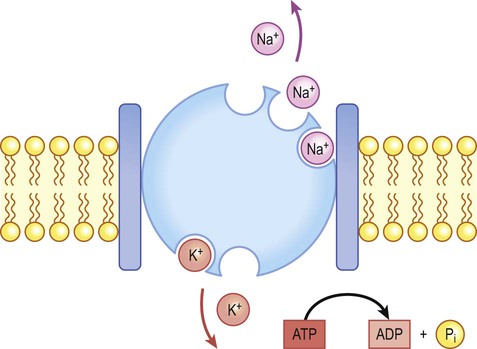
Na+/K+-ATPase (‘the sodium pump’) is responsible for maintaining the intracellular and extracellular sodium and potassium gradients in the long term.
Ionic basis of the resting membrane potential
Calculating the equilibrium potential
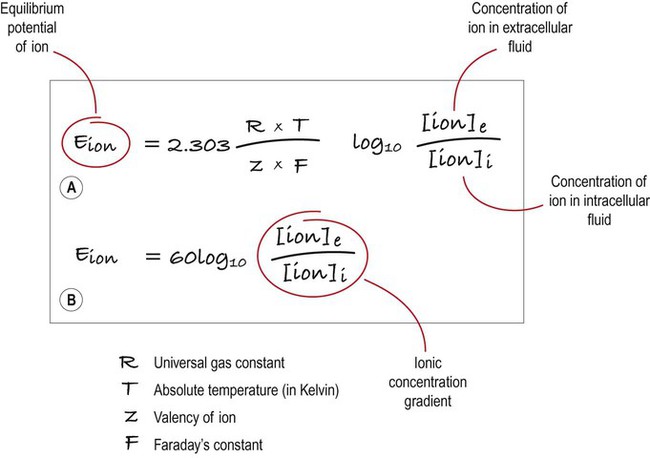
(A) This equation is used to calculate the equilibrium potential for any particular ion, taking into account variables such as temperature and ionic charge; (B) This shows exactly the same expression, but the numerical part of the equation has been simplified. Once this has been done, it is clear that the main determinant of the equilibrium potential for a particular ion is the concentration gradient (in other words: the difference in ionic concentration between the inside and outside of the cell).
Effect of membrane permeability
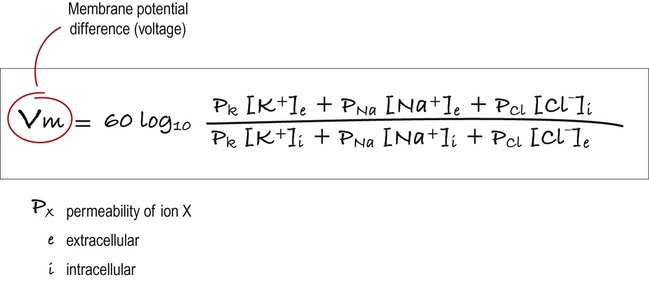
This equation combines the equilibrium potentials for each of the main ions (determined from the Nernst equation) in a single expression. The relative permeability of each ionic species is also factored in (e.g. if the membrane were mostly permeable to one particular ion, then the membrane potential would be closer to the equilibrium potential for that ion; if there were two main ionic species of equal permeability, the membrane potential would be halfway between the two equilibrium potentials).
The reversal potential
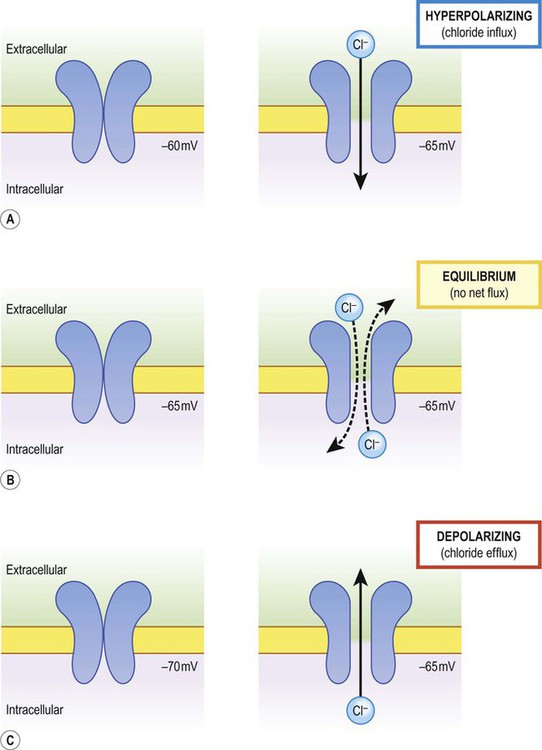
The net direction of ion flux through a membrane channel depends on the initial membrane potential of the cell and the reversal potential of the particular ion. Increasing ion conductance by opening additional channels may therefore (A) hyperpolarize the cell; (B) have no net effect on the membrane potential; or (C) depolarize the cell [see text for explanation].
![]()
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree