Fig. 1
Connection structure of neural networks
The global connected neural network is composed of 15 neurons in Fig. 1. To understand the energy coding mode in the neural network subject to stimulation, the global coupled neural network is only composed of 15 neurons in Fig. 3. The neurons are coupled with bidirectional asymmetry coupling strengths. For example, the 1th neuron is coupled to the 2th neuron with coupling strength 0.15, and the 2th neuron is coupled to 1th neuron with coupling strength 0.22. A synaptic coupling strength between neurons is uniformly distributed in the statistical sense [13], we assume that a synaptic coupling strength is uniformly distributed in [0.1 0.3].
Let coupling strength matrix: w i,j s coupling strength when the ith is coupled to jth.
The network operate as follows:

![$$ {I}_{m,j}(t)=\left\{\begin{array}{l}{i}_{m1}\,+{\displaystyle \sum_{j=1}^n\left[{i}_{0m}\left(j-1\right) \sin \left({\omega}_m\left(j-1\right)\left({t}_j-{t}_{j-1}\right)\right)\right]}\\ {}\begin{array}{cc} \begin{array}{ccc} & & \ \ \ +{i}_{0m}(n)\; \sin \left({\omega}_m(n)\left(t-{t}_n\right)\right) \end{array} & \begin{array}{cc} if & \end{array}{S}_j(t)> th \end{array}\\ {}\begin{array}{clc} {i}_{m1}+{i}_{0m}(n) \sin \left({\omega}_m(n)\left(t-{t}_n\right)\right) & & \begin{array}{cc} if & \end{array}{S}_j(t)< th \end{array}\end{array}\right. $$](/wp-content/uploads/2016/09/A315578_1_En_52_Chapter_Equ2.gif)
we treat I m,j (t) as the stimulation of the neuron, then we can obtain action potential and power consumed during action potential from the model proposed by [18]

(1)
![$$ {I}_{m,j}(t)=\left\{\begin{array}{l}{i}_{m1}\,+{\displaystyle \sum_{j=1}^n\left[{i}_{0m}\left(j-1\right) \sin \left({\omega}_m\left(j-1\right)\left({t}_j-{t}_{j-1}\right)\right)\right]}\\ {}\begin{array}{cc} \begin{array}{ccc} & & \ \ \ +{i}_{0m}(n)\; \sin \left({\omega}_m(n)\left(t-{t}_n\right)\right) \end{array} & \begin{array}{cc} if & \end{array}{S}_j(t)> th \end{array}\\ {}\begin{array}{clc} {i}_{m1}+{i}_{0m}(n) \sin \left({\omega}_m(n)\left(t-{t}_n\right)\right) & & \begin{array}{cc} if & \end{array}{S}_j(t)< th \end{array}\end{array}\right. $$](/wp-content/uploads/2016/09/A315578_1_En_52_Chapter_Equ2.gif)
(2)
where S j (t) is the sum of the stimulations for the jth neuron at time t; Q(t − τ) = [Q 1(t − τ), Q 2(t − τ), …, Q j (t − τ), … Q n (t − τ)] indicates firing states of the neurons, which take value 0 at resting and 1 at firing.
3 Energy Coding Under Different Stimulus
3.1 Instantaneous Stimulus
We assume that Topological relationships between neurons are identical for a network fully connected, stimulation the neurons receiving stimulation may be an arbitrary position in the network. We assume that the 1th, 2th, 3th neurons stimulated at the same time, set it the first neuronal group. Stimulating time is 0.1 ms, stimulating voltage is 40 mV. Then, the stimulus is immediately disconnected. The coupling strength between neurons is uniformly distributed in [0.1 0.3], the transform delay is 2 ms, refractory period is set to 0.5 ms.
With the models and parameters mentioned above, the numerical simulation result can be achieve. Figure 2 shows the power consumed by the overall neural networked.
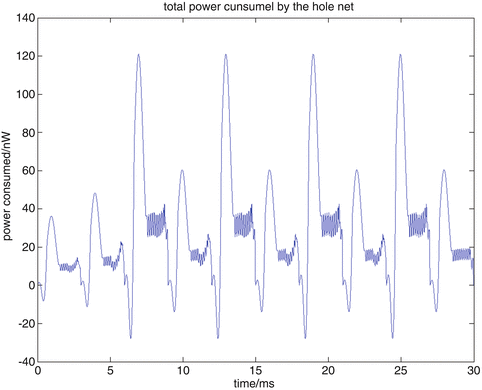

Fig. 2




The total power during the network operation
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree







