Fig. 1
A two domain-model for ion concentration dynamics in the intra- and extracellular space. The ICS is represented as a cylindrical cable (I), coated by ECS (E). The geometry is specified by three parameters, where a I is the cross section area of the cable, a E is the cross section area of the ECS and O M is the circumference of the cable. The concentration of ion species k is denoted [k] n where n represents domain I or E. Ionic movement is described by the transmembrane flux density (j kM ) and the longitudinal flux densities due to electrical migration (j kn f ) and diffusion (j kn d )
2.1 Particle Conservation
We consider the continuity equations for an ion species k with valence z k in domains I and E:
![$$\displaystyle\begin{array}{rcl} \frac{\partial j_{kI}(x,t)} {\partial x} + \frac{O_{M}} {a_{I}} j_{kM}(x,t) + \frac{O_{M}} {a_{I}} j_{kI}^{in}(x,t) + \frac{\partial [k]_{I}(x,t)} {\partial t} & =& 0{}\end{array}$$](/wp-content/uploads/2016/09/A315578_1_En_50_Chapter_Equ1.gif)
![$$\displaystyle\begin{array}{rcl} \frac{\partial j_{kE}(x,t)} {\partial x} -\frac{O_{M}} {a_{E}} j_{kM}(x,t) + \frac{O_{M}} {a_{E}} j_{kE}^{in}(x,t) + \frac{\partial [k]_{E}(x,t)} {\partial t} & =& 0,{}\end{array}$$](/wp-content/uploads/2016/09/A315578_1_En_50_Chapter_Equ2.gif)
with the sealed-end boundary conditions (n = I or E):
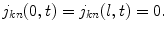
Here a I and a E are the cross sections of the ICS and ECS, respectively, and O M is the circumference of the membrane. The longitudinal flux densities are given by the generalized Nernst-Planck equation (to keep notation short, we skip the functional arguments (x, t) from here on):
![$$\displaystyle{ j_{kn} = -\frac{D_{k}} {\lambda _{n}^{2}} \frac{\partial [k]_{n}} {\partial x} -\frac{D_{k}z_{k}} {\lambda _{n}^{2}\psi } [k]_{n}\frac{\partial v_{n}} {\partial x}, }$$](/wp-content/uploads/2016/09/A315578_1_En_50_Chapter_Equ4.gif)
where the first term on the right represents the diffusive flux density (j kn d ) and the last term is the flux density due to ionic migration in the electrical field (j kn f ). The effective diffusion constant 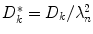 is composed of the diffusion constant D k in dilute solutions and the tortuosity factor λ n , which summarizes the hindrance imposed by the cellular structures [2, 10]. We use
is composed of the diffusion constant D k in dilute solutions and the tortuosity factor λ n , which summarizes the hindrance imposed by the cellular structures [2, 10]. We use 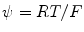 , where R is the gas constant, T the absolute temperature, and F is Faraday’s constant. The formalism we derive is general to the transmembrane flux density (j kM ), as long as j kM is a local function of v M , ionic concentrations in I and E, and possibly some additional local state variables. The formalism can be combined with any external input (j kn in ) which fulfills the constraint:
, where R is the gas constant, T the absolute temperature, and F is Faraday’s constant. The formalism we derive is general to the transmembrane flux density (j kM ), as long as j kM is a local function of v M , ionic concentrations in I and E, and possibly some additional local state variables. The formalism can be combined with any external input (j kn in ) which fulfills the constraint:
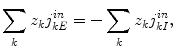
as we shall explain later.
![$$\displaystyle\begin{array}{rcl} \frac{\partial j_{kI}(x,t)} {\partial x} + \frac{O_{M}} {a_{I}} j_{kM}(x,t) + \frac{O_{M}} {a_{I}} j_{kI}^{in}(x,t) + \frac{\partial [k]_{I}(x,t)} {\partial t} & =& 0{}\end{array}$$](/wp-content/uploads/2016/09/A315578_1_En_50_Chapter_Equ1.gif)
(1)
![$$\displaystyle\begin{array}{rcl} \frac{\partial j_{kE}(x,t)} {\partial x} -\frac{O_{M}} {a_{E}} j_{kM}(x,t) + \frac{O_{M}} {a_{E}} j_{kE}^{in}(x,t) + \frac{\partial [k]_{E}(x,t)} {\partial t} & =& 0,{}\end{array}$$](/wp-content/uploads/2016/09/A315578_1_En_50_Chapter_Equ2.gif)
(2)
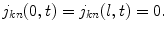
(3)
![$$\displaystyle{ j_{kn} = -\frac{D_{k}} {\lambda _{n}^{2}} \frac{\partial [k]_{n}} {\partial x} -\frac{D_{k}z_{k}} {\lambda _{n}^{2}\psi } [k]_{n}\frac{\partial v_{n}} {\partial x}, }$$](/wp-content/uploads/2016/09/A315578_1_En_50_Chapter_Equ4.gif)
(4)
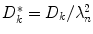 is composed of the diffusion constant D k in dilute solutions and the tortuosity factor λ n , which summarizes the hindrance imposed by the cellular structures [2, 10]. We use
is composed of the diffusion constant D k in dilute solutions and the tortuosity factor λ n , which summarizes the hindrance imposed by the cellular structures [2, 10]. We use 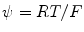 , where R is the gas constant, T the absolute temperature, and F is Faraday’s constant. The formalism we derive is general to the transmembrane flux density (j kM ), as long as j kM is a local function of v M , ionic concentrations in I and E, and possibly some additional local state variables. The formalism can be combined with any external input (j kn in ) which fulfills the constraint:
, where R is the gas constant, T the absolute temperature, and F is Faraday’s constant. The formalism we derive is general to the transmembrane flux density (j kM ), as long as j kM is a local function of v M , ionic concentrations in I and E, and possibly some additional local state variables. The formalism can be combined with any external input (j kn in ) which fulfills the constraint: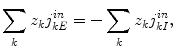
(5)
With N ion species, Eqs. 1 and 2 (with j kn , j kM and j kn in as described above) represent a system of 2N + 3 variables which are functions of x and t. These are the 2N concentration variables ([k] n for 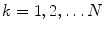 and n = E, I), and the three additional variables (
and n = E, I), and the three additional variables (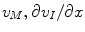 and
and 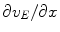 ) occurring in the expressions for the flux densities. We now seek to express
) occurring in the expressions for the flux densities. We now seek to express 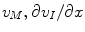 and
and  as functions of ionic concentrations, so that Eqs. 1 and 2 constitute a fully specified (and numerically solvable) system of equations.
as functions of ionic concentrations, so that Eqs. 1 and 2 constitute a fully specified (and numerically solvable) system of equations.
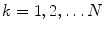 and n = E, I), and the three additional variables (
and n = E, I), and the three additional variables (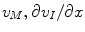 and
and 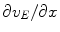 ) occurring in the expressions for the flux densities. We now seek to express
) occurring in the expressions for the flux densities. We now seek to express 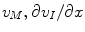 and
and  as functions of ionic concentrations, so that Eqs. 1 and 2 constitute a fully specified (and numerically solvable) system of equations.
as functions of ionic concentrations, so that Eqs. 1 and 2 constitute a fully specified (and numerically solvable) system of equations.2.2 Voltage Expressions
To reduce the number of independent variables to the 2N state variables ([k] n ) we use three additional constraints:
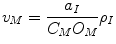
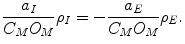
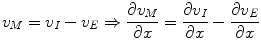
Equation 6 is the assumption that the membrane is a parallel plate capacitor. Then v M is determined by the density of charge on the inside of the membrane, which in turn is determined by the ionic concentrations:
![$$\displaystyle{ \rho _{n} = F\sum _{k}z_{k}[k]_{n} +\rho _{sn}. }$$](/wp-content/uploads/2016/09/A315578_1_En_50_Chapter_Equ9.gif)
For practical purposes, we have included a density of static charges (ρ sn ) in Eqs. 6 and 7, representing contributions from ions that are not considered in the conservation equations. If the set [k] n include all present species of ions, then ρ sn = 0.
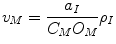
(6)
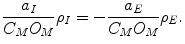
(7)
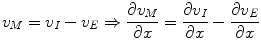
(8)
![$$\displaystyle{ \rho _{n} = F\sum _{k}z_{k}[k]_{n} +\rho _{sn}. }$$](/wp-content/uploads/2016/09/A315578_1_En_50_Chapter_Equ9.gif)
(9)
Stay updated, free articles. Join our Telegram channel

Full access? Get Clinical Tree






