in Two-Coupled Cells Network in the Pre-Bötzinger Complex

(1)

$\displaystyle\begin{array}{rcl} \dot{h}_{i} =\varepsilon (h_{\infty }(v_{i}) - h_{i})/\tau _{h}(v_{i}),& &{}\end{array}$

(2)

$\displaystyle\begin{array}{rcl} \dot{n}_{i} = (n_{\infty }(v_{i}) - n_{i})/\tau _{n}(v_{i}),& &{}\end{array}$

(3)

$\displaystyle\begin{array}{rcl} \dot{s}_{i} = \alpha _{s}(1 - s_{i})s_{\infty }(v_{i}) - s_{i}/\tau _{s},& &{}\end{array}$

(4)

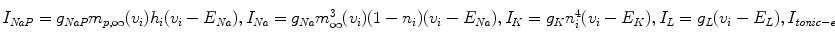where i, j = 1, 2 and i ≠ j. C represents the whole cell capacitance. I _Na,I _Kand I _Lare a fast Na ⁺, delayed-rectifier K ⁺, passive leakage current, respectively. I _syn−erepresents a excitatory synaptic input from other cell in the network. Specifically, $I_{\mathit{NaP}} = g_{\mathit{NaP}}m_{p,\infty }(v_{i})h_{i}(v_{i}-E_{\mathit{Na}}),I_{\mathit{Na}} = g_{\mathit{Na}}m_{\infty }^{3}(v_{i})(1-n_{i})(v_{i}-E_{\mathit{Na}}),I_{K} = g_{K}n_{i}^{4}(v_{i}-E_{K}),I_{L} = g_{L}(v_{i}-E_{L}),I_{\mathit{tonic-e}} = g_{\mathit{tonic-e}}(v_{i}-E_{syn-e}),I_{\mathit{syn-e}} =\sum \limits _{i\neq j}g_{\mathit{syn-e}}s_{j}(v_{i} - E_{\mathit{syn-e}})$ and $I_{\mathit{CAN}} = g_{\mathit{CAN}}f([Ca]_{i})(v_{i} - E_{\mathit{Na}}).$

The activation of the CAN current by the calcium concentration is given as $f([Ca]_{i}) = (1 + (K_{\mathit{CAN}}/[Ca]_{i})^{n_{\mathit{CAN}}})^{-1}.$ The calcium dynamics is described as $d[Ca]_{i}/dt = f_{m}(J_{ER_{\mathit{IN}}} - J_{ER_{\mathit{OUT}}})$ , $dl_{i}/dt = AK_{d}(1 - l_{i}) - A[Ca]_{i}l_{i}$ , in which $J_{ER_{\mathit{IN}}} = (L_{IP_{3}} + P_{IP_{3}}[ \frac{IP_{3}[Ca]_{i}l_{i}} {(IP_{3}+K_{l})([Ca]_{i}+K_{a})}]^{3})([Ca]_{\mathit{ ER}} - [Ca]_{i})$ , $J_{ER_{\mathit{OUT}}} = V _{\mathit{SERCA}} \frac{[Ca]_{i}^{2}} {K_{\mathit{SERCA}}^{2}+[Ca]_{i}^{2}}$ and $[Ca]_{\mathit{ER}} = \frac{[Ca]_{Tot}-[Ca]_{i}} {\sigma }.$ The meaning and values of other parameters are same as that in [10].

3 Bursting and Pattern Transition Mechanisms

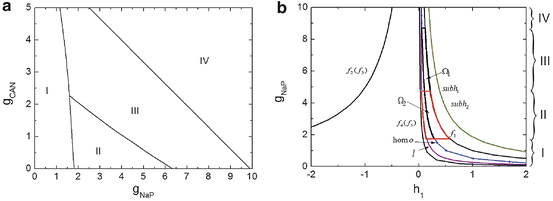
The activity patterns depending on parameters g _NaPand g _CANare illustrated, as shown in Fig. 1a, The horizontal axis represents g _NaPand the vertical g _CAN. Two-coupled cells in the pre-BötC can generate two types of oscillations: the in-phase and anti-phase oscillations [1], so the two-parameter space can be divided into four regions: region I (silence), region II (in-phase bursting and anti-phase bursting), region III (in-phase bursting and anti-phase spiking) and region IV (in-phase spiking and anti-phase spiking). We chose g _CAN = 0. 7 nS as a representative and explore the bursting transition mechanisms between these regions with g _NaPchanging. The systems yield a steady calcium concentration $[Ca]_{1} = [Ca]_{2} = 0.02104057\,\mathrm{mV}$ with $IP_{3} = 0.8\,\upmu \mathrm{M}$ . 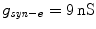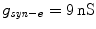h ₁ and h ₂ are almost equal when $g_{\mathit{syn-e}} = 9\,\mathrm{nS}$ [1], we can consider h ₁ and h ₂ as one single slow variable, h ₁. The two-parameter bifurcation analysis is shown in Fig. 1b. As g _NaPincreases, the systems undergo regions I, II, III and IV. The maximum and minimum values of limit cycles in the full system (1)–(4), we named “the slow variable regions” Ω ₁ and Ω ₂ [8], are appended in Fig. 1b. The bifurcation curves in regions Ω ₁ and Ω ₂ are different which play an important role in determining which types of bursting or spiking can occur.